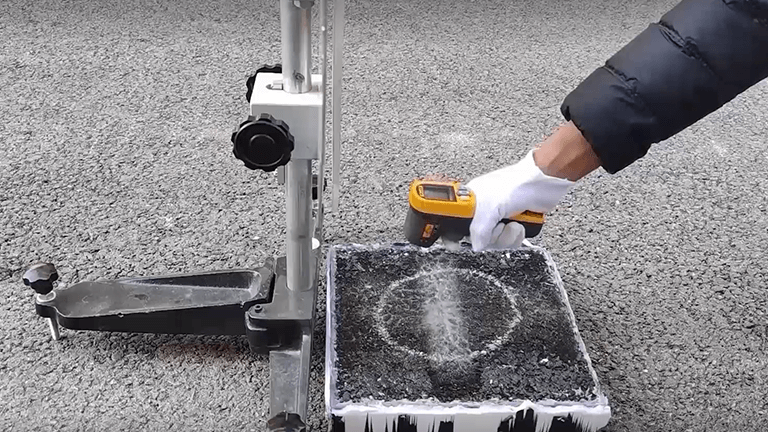
This video is aimed to show the process of determining the coefficient of friction of an icy road surface. Using the pendulum friction coefficient meter. Ice on the road surface can lead to a significant reduction in the coefficient of friction and thus endanger driver safety. We want to establish a complete and scientific experimental procedure indoors to determine the coefficient of friction of icy road surfaces. A pendulum friction tester was used to conduct in the experiment and asphalt slabs and water layers were frozen simultaneously to simulate road icing. Different levels of snowfall was also simulated on asphalt slab with varying ice thickness to make the results more intuitive. Preparation of the equipment. First, the pendulum friction coefficient meter, during the experiment ensure that B P T is within its surface life and that surface is clean and undamaged. Its components are base, leveling spiral, leveling bubble, pointer, pendulum, lifting spiral, fastening spiral, huddle, and dial. Then the asphalt slab, ensure that asphalt mixture sample size use for experiment is 30 centimeters by 30 centimeters by five centimeters. The right figure shows the asphalt slab wrapped with molds. Next the freezing equipment, ensure that this equipment used in the experiment can freely regulate the temperature between subzero 20 centigrade to zero centigrade. Other equipment used in the experiment includes, tripod, measuring cylinder, rubber sheet, pavement thermometer, sliding length ruler and brush. The size of the rubber sheet used in the experiment is 6.35 millimeters by 25.4 millimeters by 76.2 millimeters and should meet the quality requirements given in the following table. During the experiment, ensure that the rubber sheet has no following defects:First, there are oil stains;Second, rubber sheet width edge wear is greater than 3.2 millimeters. Third, rubber sheet length direction wear is greater than 1.6 millimeters. Before using a new rubber sheet, ensure that the rubber sheet is measured 10 times on a dry surface before employed for official testing. Calculation and analysis of snowfall the table gives the snowfall class classification to consider extreme cases takes 24 hours of snowfall at the condition for the study. Moreover, to ensure the ease of experimentation the upper limits of each level of snowfall division as used for the corresponding calculation and analysis. After calculation, different levels of snowfall test at the corresponding water volume of the samples as provided in the table. Experiment does not consider the influence of the extraordinary snow storms and number the very light snow to big blizzard from 1 to 6. Equipment calibration Place the BPT in a suitable position. A suitable position means that the ground is flat and free of potholes, rotate the leveling spiral on the base of the BPT to keep the leveling bubble in the middle position. Loosen the fastening spiral, rotate the lifting spiral to make the pendulum lift and swing freely. Then tighten the fastening spiral. Place the pendulum arm on the right cantilever of the pendulum table keeping the arm in the risen toe position while rotating the pointer to the right side flush with the arm. Press the release button to let the pendulum arm swing freely and then the pendulum crosses the lowest point. To reach the highest point, hold it by hand. The pointer should indicate zero at this time. If the pointer does not show the zero point loosen and tighten the narrowing nut to make it show the zero point. Place the asphalt slab directly under the pendulum while loosening the fastening spiral so that the lowest edge of the rubber sheet touche the surface of the asphalt slab. Prepare the sliding length ruler and bring it close to the rubber sheet. Lift the carrying handle to make the left scale mark of the sliding length ruler flush with the lowest edge of the rubber sheet. Lift the carrying handle and move the pendulum to the right again so that the lowest edge of the rubber sheet only touches the surface of the asphalt slab. Observe further, the sliding length jeweler is flush with the edge of the rubber sheet. If flush, the sliding length meets a requirement of 136 millimeters. Otherwise, continue the following operation. Turn the lifting spiral to adjust the height of the pendulum to make the sliding length meet the requirements. Vital tan is needed. Twist the level spiral on the base. The level in bubble needs to remain centered during an adjustment. Friction coefficient determination. Select seven pieces of asphalt slabs. Clean their surface with a brush and dry naturally at room temperature. Number the asphalt slabs in turn place the asphalt slabs into modes and simultaneously coup and freeze with the water layer. The sevens samples were placed into the freezer at a controlled temperature in a sub zero 10 centigrade for 24 hours. After freezing, turn down the samples in turn, remove the modes and place them on the BPT centers, which have been leveled and zero use the pavement centimeter to measure the surface temperature of the sample and record, perform sliding length calibration. To ensure sliding distance of 126 millimeters press the pendulum arm release switch and when the pad arm crosses the lowest point and swings to the highest one held by hand, read and recorded restore both the pendulum arm and the pointer to the zero and horizon positions respectively. The sliding length should be re calibrated each time a new sample is tested. Repeat the steps for 10 times. Measure seven samples in sequence. Each sample has 10 measurement results and both the minimum and the maximum value difference should be less than three. Data analysis recorded data in the table and the average measurement results to get average values bring the temperature value measured into the equivalent. To obtain the temperature compensated value subtract the compensated BPN value from average BPN value in the table to obtain the final temperature compensated BPN value plot the final BPN values in table four and the bar graph for more intuitive results. Representative results:When comparing sample seven and the other six groups, I think it is observed to significantly reduce the friction coefficient of the pavement. Based on sample one very light snow force is not to have a serious impact on the road friction coefficient. With respect to sample two, three, and four the surface friction coefficient was observed to gradually decrease for sample four, five and six. The average final BPM values are identical. This may indicate that the road friction coefficient of the ice layer tends to stabilize and another measure of a thicker ice layer is not necessary. The bar graph can better reflect the wide ratio of friction coefficient. Sample one represents very light snow that adhere to the pavement surface after icing resulting the reduction in the pavement friction coefficient and its BPM value decreased by approximately 43%compared to the dry sample. Sample two, three and four correspond to light medium and heavy snow respectively. Among them the BPM value of medium snow is only one half that of light snow because the thickness of the iced layer corresponding to little snow is of like two minimum. The micro structure of the sample surface still affects the friction coefficient value and the BPN is larger. The BPNs of the heavy snow, blizzard and large blizzard samples are the same, which may stop an ice thickness reaches 11 minimum. The rubber sheets can no longer deformed the ice layer by compacting it. Conclusion:The friction coefficients of seven asphalt slabs were determined by means of a pendulum friction coefficient tester, and the final results were corrected for temperature. It can be seen that, the effect of pavement icing on the coefficient of friction is indeed significant, with even slightly light snow resulting in patches of ice reducing the pavement of friction coefficient by about 40%The final results can provide a reference for road design and winter pavement maintenance.
发布时间:2023-01-06 JOVE
Anton Astner:This protocol describes the preparation of engineered micro and nanoplastics sourced from a wide range of polymer feed stocks, such as pellets and films representing authentic model materials. This method efficiently forms micro nanoplastics through a simple mechanical procedure involving cryogenic milling, seething and wet grinding that can serve a surrogate materials for environmental studies. To begin retrieve foam from roll and cut P B A T foam into strips with a paper cutter pre soak fragments in deionized water for 10 minutes and transfer the foam material into a cryogenic container. Slowly add 200 milliliters of liquid nitrogen to a cryogenic container transfer pres soaked foam fragments or pellets carefully into the cryogenic container with steel tweezers. Next, transfer frozen foam fragments or pellets into a blender and process for 10 seconds. Add 400 milliliters of DI water and blend the foam water slurry for five minutes. Transfer slur into a buchner funnel with a filter and apply vacuum for one hour. Then transfer the sample with the funnel into a vacuum oven. Dry at 30 degrees Celsius for at least 48 hours. Weigh polymer film or pellet samples and transfer into a 50 milliliter glass jar. Place the rectangular delivery tube with a 20 mesh seed in the slot in front of the rotary cutting milk and raise the delivery tube until it hits the stock pin. Position the glass plate over the milling chambers face and secure it with the adjustable clamp. Next place a 50 milliliter glass jar under the mill outlet. Position the sliding side arm support on the mill slightly off center on the front glass and tighten with the neural bolt. Insert the hopper funnel on top of the mill into the opening of the upper milling chamber. Plug a line cord into a power outlet and press the cord switch to start the mill operation. Feed the sample slowly and add the next batch of film or pellet fragments after the audible noise reduces. After processing the film or pellet fragments press the cord switch to stop the mill operation for approximately 20 minutes to cool down. Clean the cutting chamber with a spatula and bristle brush And collect particles in the glass jar. Remove the 20 mesh 840 micrometer delivery tube and replace it with the 60 mesh delivery tube. Upon completion of the first batch reintroduce the collected material into the mill hopper. Follow the same procedure for the 60 mash milling fraction. Recover the remaining particles in the chamber and add them to the collected main fraction. Prepare a slurry of microplastics. Start by taking di water into a glass beaker and insert a stirring bar. Introduce eight grams of the collected 250 micrometer plastic fraction. Place the glass beaker on a stirring plate and stir magnetically for 24 hours at 400 rotations per minute. To soak the particles in water transfer the particles into a plastic container. Fill in additional two one liter plastic containers with di water, which will be used to rinse off endearing particles on the grinder's hopper. During the grinding process. Place stones with a 46 green size in the wet friction grinder and fasten the center nut. Hand tight with a 17 millimeter wrench add the hopper on top and fasten the four bolts with the five millimeter Allen wrench. Place a one liter plastic collection jar under the outlet of the collider. Place a second empty one liter bucket next to the outlet which will be used for exchanging while processing. Switch the power on and carefully adjust the gauge clearance by turning the adjustment wheel clockwise, corresponding to a positive 0.10 micrometer shift from the zero position until hearing the grinding stones touch. Next, adjust the flexible measurement ring to zero and turn the wheel counterclockwise immediately. By default, the speed is adjusted to 1500 revolutions per minute. Turn the adjustment wheel clockwise until the stones touch and gently fill the water nanoplastic slurry into the hopper. Decrease the gap continually to a clearance gauge of negative 2.0 corresponding to a negative 0.20 micrometer shift from the zero position after the slurry was introduced. Collect the slurry by exchanging the collection buckets. Once the filling level and the bucket exceeds 0.5 liters collect and reintroduce the particles into the grinder between 30 to to 60 times higher. Passes result in smaller particle size. Wash endearing particles on the hopper with the prepared DI water bottle to allow suitable slurry mixing while processing. Recover the slurry and stir for four hours at 400 rotations per minute at 25 degrees Celsius to allow it to mix well let the slurry stand for 48 hours to stabilize. The numerical analysis revealed the bimodal particle size distribution for nanoplastics produced from both feed stocks. The main particle populations for nanoplastics from P B A T pallets were at approximately 79 and 530 nanometers, and corresponding number density frequency values were at 25 and 5%respectively. On the other hand, nanoplastics derived from P B A T foams possessed size maxima at approximately 50 and 106 nanometers with corresponding number density frequency values of 11 and 10%respectively. FTIR R results for P B A T Nanoplastics indicate a distinct decrease in absorbance values between 980 and 1200 centimeter inverse reflecting the starch component leaching during wet grinding. Consistent with previous study observations Anton Astner:For microplastic formation from plastic film and pellets cryogenic pretreatment induces embrittlement to the plastics. It mimics the impact of environmental weathering but not perfectly, which allows accelerated defragmentation. The cryo milling procedure combined with the wet grinding process allows the processing of numerous differently plastic feed stocks to form authentical micro nanoplastics used in environmental studies studies.
发布时间:2022-07-27 JOVE
Traditionally we've used the tester to study non-living tissues to calculate friction coefficients but we are significantly expanding the tester's capabilities to test living tissues and to evaluate biological interactions. The friction testing device delivers reciprocal translating motion and compressive load to contacting surfaces of living tissue explants. The device is modular, allowing for the testing of various biological counterfaces. The method could provide insight into how frictional forces affect the mechanical and mechanicobiological responses of living cartilage and synovium, which may lead to new strategies for maintaining joint health. Begin by harvesting the juvenile bovine synovium. Using a scalpel blade, trace the outline of the synovium region of interest. Using forceps, grasp one end of the synovium and gently lift to stretch the synovium distal to the underlying bone. Use a scalpel blade to remove the synovium from the bone and place the tissue in appropriate culture media or testing bath solution. Then harvest the juvenile bovine cartilage by securing the tibia in an adjustable holder. Remove the meniscus carefully while avoiding contact with the cartilage surface. On the outer edges of the tibial plateau, use a box cutter to cut perpendicular to the cartilage toward the bone. Cut completely through the cartilage to make straight edges or sides. Remove excess tissue. On the outside edges, use the box cutter to make a clean cut at the interface between the bone and the cartilage. To remove the tibial strip from the plateau surface, gently insert a flathead screwdriver below the cut and gently rotate to loosen the articular cartilage from the subchondral bone. As the sample loosens, slowly push the screwdriver forward until the cartilage strip detaches from the bone. Ensure that the screwdriver is pushed towards the bone, not the cartilage. Using a box cutter, cut the tibial plateau surface to produce rectangular samples of desired size and thickness. Place tissue in appropriate culture media or testing bath solution. If the tibial strip is used as the bottom counterface, remove the detachable magnetic base and glue a 60-millimeter diameter Petri dish to the top surface of the detachable base. With the Petri dish glued in place, attach the detached base to the fixed base and mark the Petri dish to indicate a sliding direction. Apply a small amount of cyanoacrylate to the center of the dish. Align the tibial strip with the sliding direction of the stage. Gently press the cartilage strip onto the dish. Restore the removable magnetic base to its paired fixed base in the friction tester. Fill the Petri dish with the desired testing bath solution. If the synovium is used as the top counterface, remove the loading platen and support rod from the friction tester. Place the synovium on top of the circular platen. To secure the synovium, spread an O-ring over its circumference. Using forceps, gently pull at the synovium to stretch tissue taut and flat beneath the O-ring. Trim excess tissue with surgical scissors. Restore the loading platen and support rod to the friction tester. Adjust the vertical height of the loading platen such that the synovium hovers over the bottom counterface and is submerged in the testing bath. Insert the mounted specimens into the friction tester device. Open the analog data build MF DAQ. Initialize Load PID and Trigger Dynamic Caller Windows in the program. Run the analog data build MF DAQ and initialize load PID window by pressing the Run button. Navigate to the Stepper tab in the Trigger Dynamic Caller window. Specify the acceleration, speed, and distance of the translation stage in the user input boxes. To input the test duration, click on the Open Folder button at the bottom right of the Time State table and select the Stepper Time Index file path. Then specify the test duration again in the Voice Coil tab. Select the Voice Coil Index file path by clicking on the Open Folder button at the bottom right of the Time State table and select the file. This must be performed whether the voice coil is used or not. Apply the normal load. If using deadweights, place desired weights on the linear bearings above the loading platen. Ensure that the load applied plus the weight of the loading platen and support rod do not surpass the load cell-rated capacity. Select the path and file name for data storage using the Open Folder button to the right of the Right to File box. Save the file with a txt extension. Center the bottom counterface underneath the top counterface and set this as the zero X position. To do this, run the Trigger Dynamic Caller window by pressing the Run button. In the Stepper tab click on the Home button to move the stage to the last saved zero X position. If counterfaces are not aligned, move the stage by clicking the green left and right arrow buttons. When the desired location is reached, click on the zero button to save the current stage location as the new zero X position. Stop the Trigger Dynamic Caller window by clicking on the Stop button. Once the top and bottom counterfaces are centered, initiate friction testing of the samples by starting the cyclic movement of the stage. Once the stage moves, slowly bring the top counterface into contact with the bottom. Let the test run, collecting the friction testing data. After the desired testing duration, stop the test by pressing the Stop button and by unloading the specimens by raising the top counterface and moving it out of contact with the bottom counterface. Use the custom code to calculate the friction coefficient and the hysteresis per cycle. Ensure a single folder contains all relevant files. Open the Friction Cycle run. m file. Click on the Run button in the script. Select the raw data file to analyze and the desired save location. The friction coefficient and hysteresis plots will be output by MATLAB. A synovium-on-cartilage configuration was used to friction test juvenile bovine explants where the synovium was mounted on a 10-millimeter diameter acrylic loading platen such that the intimal layer would be in contact with the underlying cartilage. A tibial strip was used as the cartilage counterface. An effective friction coefficient was calculated from the average of FT divided by FN over each reciprocating cycle and then plotted against test duration to yield a friction coefficient-versus-time plot. For each test, friction coefficient values were averaged over the entire test. In a PBS testing bath, the average friction coefficient values increased as the contact stress increased. Conversely, the average friction coefficient values remained similar as the contact stress increased in a bovine synovial fluid bath. Remember to start collecting load data before bringing the surfaces into contact. This ensures a proper tare load can be calculated. Tissue and lubricating bath components can be assessed before and after testing to evaluate the biological changes imparted by a given experimental regimen.
发布时间:2022-06-02 JOVE
The overall goal of this procedure is to test the frictional properties of phyllosilicates, with faults sheared in the in-situ geometry, and to show that this friction is significantly lower than friction of powders obtained by the same material. During the long-term evolution of tectonic faults, numerous geological studies have documented fluid-assisted reaction softening, that promotes the replacement of strong and granular minerals with phyllosilicates. In particular, fracturing processes along faults increase permeability, and facilitate the influx of hydrous fluids into the fault zone. Fluids react with fine-grained rock, promoting dissolution of the strong minerals like quartz, feldspar and calcite. They become platy phyllosilicates and form foliated microstructures, like the one presented here in green. Slip along the phyllosilicates from the micro-scale is transmitted to the entire fault zone via the interconnectivity of the phyllosilicate-rich shear zones. This is an example of the continuity of the phyllosilicate shear zone at the outcrop scale, that can be extended up to crustal-scale faults with thicknesses of more than 100 meters. Along phyllosilicate-rich fault like this one, the tonic shearing has produced the phyllosilicate alignment, producing this fault rock anisotropy. In order to take into account the role of anisotropy in frictional properties of the fault, we have to collect the right rock samples. To do that, we have to collect a representative rock sample, and within the outcrop, we select a portion where the kinematic indicators are best preserved. And then we use a chisel and a hammer to collect the rock sample. Once the rock sample has been collected, we mark the sense of shear, and then we bring the rock sample to the lab for the experiment. With this procedure, we cut the rock samples to obtain wafers that fit the forcing blocks of the rock deformation apparatus. This is usually achieved in 2 steps. In the first step, we use a standard laboratory saw to obtain rock samples that are slightly larger than the forcing blocks. Secondly, we use a high precision rotary blade, or a hand grinder, to shape the wafers so they're 5 by 5 centimeters in area, and about 1 centimeter in thickness. From the same piece of rock, we use a disk mill to obtain a granular material that is sieved to reach the desired grain size, usually below 125 microns. The 2 identical wafers are mounted on stainless steel forcing blocks with a nominal frictional area of contact of 5 by 5 centimeters, and then are assembled with a central forcing block to compose the symmetric, double-direct configuration. In the same way, the powders are used to construct 2 identical layers whose thickness is about 5 millimeters, and whose area of contact is 5 by 5 centimeters. These are then used to compose a similar double-direct shear configuration. At this point, the double-direct shear configuration is positioned within our biaxial apparatus, and we're ready to start the friction experiment. We use a servo-controlled hydraulic piston to apply and maintain a constant normal stress on the rock sample. Then by advancing the vertical ram, we apply shear stress at constant sliding velocity;it is usually 10 microns per second. Most of the experiments are characterized by an initial strain hardening, where the shear stress increases rapidly during elastic loading, followed by shear stress at steady state. The shear stress to normal stress ratio gives us the friction coefficient. At the end of the friction test, we carefully extract the experimental fault, we impregnate the rock sample with epoxy resin, we cut the sample in a direction parallel to the sense of shear, and we build thin sections from the cuts for microstructural studies. We use an optical microscope to characterize the bulk faults on the microstructure. We analyze microstructures with a scanning electron microscope to investigate the main deformation processes. We use a transmission electron microscope to obtain details about deformation processes down to the nanoscale. In a diagram of normal stress versus shear stress, both the solid foliated wafers and the powder samples plot along the line, consistent with a brittle failure envelope. But the solid wafers have a friction value significantly lower than the powdered analogs. In particular, the powders show friction of about 0.6, whereas the foliated rocks have significantly lower values. At each normal stress, the foliated rocks have a friction coefficient that is 0.2 to 0.3 units lower than the powders made from them. Microstructural studies of the tested rocks show that the low friction of the solid wafers is due to sliding along the pre-existing, very fine-grained foliations made of phyllosilicates. TEM images show that slip is mainly accommodated by fracturing, translation, and rotation along the phyllosilicates, with frequent inner layer delamination. In contrast, experiments conducted on powders indicate that much of the deformation occurs along zones effected by fracturing and grain size reduction. This results in higher values of friction. This is a summary of the frictional properties of natural, phyllosilicate-rich tectonic faults from different tectonic environments. Data show that friction is in the range of 0.1 to 0.3, and this friction is significantly lower than the traditional Byerlee value of friction obtained from a large gamut of rock types, that are predominantly made of granular mineral phases. To summarize, our friction experiments show that foliated samples are extremely weak compared to their powdered equivalents. Microstructural studies indicate that the lower friction, or in other words, fault weakness of the foliated fault rocks is due to the reactivation of the pre-existing natural phyllosilicate-rich surfaces. These surfaces are absent in the powdered samples since the sample preparation step destroys them. Our friction tests on solid foliated samples show that low friction, and therefore fault weakness, can occur in cases where weak mineral phases constitute only a small percentage of the total fault rock, implying that a significant number of crustal faults are weak.
发布时间:2021-11-06 JOVE
By following the steps of this protocol, any scientist should be able to set up the COST-jet, measure the applied power, and apply the atmospheric pressure plasma generated in the device on a solid or liquid substrate in a reproducible manner. Applying the COST-jets onto samples such as biological substrates allows direct comparison between research results of different groups, as well as direct comparison between simulation and experiment. When applied to complex systems such as biological substrates and liquids, the COST-jets can really help us simplify the problem and focus on the interesting science. Before the treatment, prepare the experimental setup by arranging the gas supply with all metal gas line. Choose the mass flow controllers used to provide the feed gas, avoiding any TPFE or similar plastics tubing. Realize the admixture of reactive gases with a system consisting of multiple mass flow controllers. For smaller admixtures, use a counter mixing unit to reduce the time needed for the mixing to complete. Add a valve between the gas supply lines and the jet. Clean the gas supply lines before the surface treatment by pumping and refilling them approximately three times. Add a pump to the system and turn it on, then switch off the pump and turn on the gas flow. Add a molecular sieve trap or cold trap. Connect the COST-jet device to a gas supply and to the power supply, then connect the integrated electrical probes to an oscilloscope. Properly compensate a commercial voltage probe. Open the COST-jet housing by removing two of the screws and connect the commercial voltage probe to the powered copper line as well as the grounded part of the jet. Next, perform a probe calibration routine. Apply a small voltage to the COST-jet and tune the variable capacitor of the LC circuit with a screwdriver to reach the optimum coupling. Perform a voltage calibration by comparing the actual voltage to the measured voltage using linear regression and calculate a calibration constant. Remove the commercial voltage probe and close the COST-jet housing. Again, apply a small voltage to the COST-jet and tune the variable capacitor of the LC circuit using a screwdriver to reach the optimum coupling. Set up a gas flow rate of approximately one standard liter per minute of helium using the mass flow controllers. Open the valve between the gas supply system and the COST-jet, then apply a low voltage to the electrodes and increase the amplitude until the plasma ignites. Allow the setup to warm up for approximately 20 minutes. Connect the oscilloscope monitoring the voltage and current applied to the COST-jet to a computer. Start the COST Power Monitor software and switch to the Settings panel. Fill in the correct channels connected to the oscilloscope and the calibration constant. Switch off the gas flow and apply a voltage that is in the typical range of voltages used for the actual operation of the discharge. Change to the Sweep panel and take a reference phase while the plasma is still off by pressing the Find button. After finding the reference phase, switch the gas back on. Press the Start and Pause button to start or pause the electrical measurements. Prior to any treatment, clean the gas supply lines, set the control parameters, and allow the setup some warmup time as previously described. For solid surface treatment, choose the distance between the COST-jet and the treated surface. Start the treatment time by either switching on the plasma or using a mechanical shutter. If necessary, check the gas flow pattern in front of the target using Schlieren imaging. For liquid treatment, pour the liquid to be treated into an adequate container, taking into consideration the influence of the gas flow on the liquid surface. Start the treatment. Avoid pressure surges on the surface of the liquid due to a sudden change in gas flow, which could cause liquid splashes into the discharge geometry. Use a mechanical shutter or slowly increase the gas flow. Take into account the mixing of the liquid due to friction between neutral gas flow and liquid surface. This protocol was used to apply the COST-jet to different surfaces and liquids. To demonstrate the reliability of the device, the electrical power in a helium plasma generated in five different COST-jet devices was measured using a gas flow of one standard liter per minute. Here, each color represents one of the devices. All devices show similar behavior with deviation originating from the uncertainty of the power measurement and microscopic differences in the setups. The etch profile of an ACH film for a three minute treatment with the COST-jet is shown here. The pattern shows a circular structure representing the cylindrical symmetry of the plasma effluent. The surface loss probability of atomic oxygen can be estimated based on etch profiles in combination with numerical simulations. Vortices in liquid are caused by gas stream impinging on the liquid surface. A laser sheet illuminating tracer particles in the liquid makes it possible to observe the trajectory and velocity of these particles via particle image velocimetry. The occurring depression of the liquid surface underneath the gas channel of the plasma jet can also be observed. The most important part of this protocol is to keep the system as reproducible as possible. This is done by accurately measuring the power as well as cleaning the gas supply lines to avoid any impurities. Using this protocol, the COST-jet can be applied to any kind of sample in a reproducible fashion.
发布时间:2020-11-02 JOVE
The significance of this protocol is that it allows an accurate rheological characterization of non-Newtonian biological fluids, such as mucus, especially when only very small sample volumes are available. This technique facilitates the characterization of the apparent yield stress and viscoelasticity of complex structured biological fluids, such as gill raker mucus. This method enables the yield stress estimation of gill raker mucus, and facilitates the rheological characterization and protocol development of similar biological fluids, such as human, animal, and plant secretions. Demonstrating the procedure with Kartik Bulusu will be Samantha Racan, a graduate student from our laboratory. To prepare approximately two milliliters of 100 milligrams per milliliter concentrated fish mucus solution, perform serial dilution of two milliliters of 400 milligrams per milliliter concentration by adding deionized water in one to two ratio twice. Place the vial of mucus solution onto a shaker until the solution is adequately homogenized and any mucus particulate agglomeration has been mitigated. To calibrate the rheometer, launch the rheometer instrument control software and select the calibration and instrument tabs. Click calibration and calibrate to ensure that the calibration values are within 10%of each other and click accept. Turn the shaft on top of the rheometer to install the cone angle geometry. The software will detect the 40 millimeter diameter, one degree, zero minute, 11 second cone angle geometry. To perform the rheometer geometry calibration, click calibration under inertia and click calibrate. Click accept to accept the calibration. Click calibration under friction and click calibrate. Click accept to accept the calibration. The geometry inertia and friction calibration values should be recorded in the appropriate units. To perform the zero gap initialization, click gap in options. In the pop-up window, set the axial force to one Newton and click OK, then click the zero gap icon. The initialization is complete when the axial force experienced by the geometry becomes greater than or equal to one Newton as it touches the Peltier plate. To ensure that the rheometer gap is zeroed so that its reference position is accurate, use the up and down arrows to raise the geometry to any arbitrary height. The control screen on the rheometer instrument and the control panel of the rheometer instrument control software will both display the gap height. To perform the rheological experiment and the linear viscoelastic range of a mucus solution of interest, load approximately 300 microliters of the fish mucus solution into a one milliliter pipette tip and load the solution onto the Peltier plate. Press the go to trim gap icon to lower the geometry onto the Peltier plate. Use the pipette to remove any excess mucus solution while applying a small angular velocity to the motor and click the stop and apply icons until the torque value reaches minimum. To perform oscillation amplitude experiments, in the procedures menu of the experiments tab, set the oscillation and amplitude and set the temperature to 22 degrees Celsius. Set the soak time to 120 seconds and check wait for temperature. Set the frequency to one Hertz, the torque to 10 to 10, 000 micronewton meters, and the points per decade to 10. Next, click the insert duplicate step icon. Change amplitude to frequency. Set the temperature to 22 degrees Celsius and set the soak time to zero seconds. Set the strain percent to one. In the logarithmic sweep menu, set the frequency to 20 to 1 hertz and the points per decade to 10. To set up the flow sweep procedure, click the insert duplicate step icon and then change oscillation to flow and select sweep to set the temperature to 22 degrees Celsius and set the soak time to zero seconds. Set the shear rate to 1 to 10, 000 per second and the points per decade to 10 and check the steady state sensing box. Press the go to geometry gap icon to lower the geometry to the preset suitable gap per specific geometry and click start in the instrument software and observe the motion of the cone geometry. A real-time plot that reports the loss in storage moduli will be generated by the rheometer in the main window after the temperature soak time is reached. Right-click to select the graph variables tab and set the x-axis of the plot to oscillation strain percentage to view the data of interest. To view the angular frequency data, right-click frequency sweep and select send to new graph. To view the apparent viscosity versus shear stress data in real time, right-click flow sweep and select send to new graph, then save the file that contains both the experimental procedure and results in the native file format of the rheometer instrument control software when the experiment ends. For graphical analysis, export the mucus rheology data into a spreadsheet and run supplemental codes for the apparent viscosity for varying shear strain rates and loss modulus, storage modulus, and phase angle for varying oscillation stresses to generate graphs of the results. As observed in dynamic oscillation amplitude data, the storage modulus of the 200 milligram per milliliter concentration decreased and crossed over the loss modulus within the stress range. The phase angle data for this concentration demonstrated a steady transition to viscoelastic liquid with a transitional region of yield stresses. Modulus data for the 400 milligrams per milliliter concentration demonstrated a yielding phenomenon with a crossover point between the storage and loss moduli that occurred at the apparent yield stress of approximately 0.27 pascals. The phase angle data for the 400 milligrams per milliliter concentration demonstrated a sharp transition to viscoelastic liquid with a crossover point at approximately 0.27 pascals. As observed in the steady shear rate data, the 100 milligrams per milliliter concentration exhibited a predominantly constant viscosity outside the highlighted regions of instrument limitation, a negligible flat region, and a Newtonian fluid-like behavior. The 200 milligrams per milliliter concentration demonstrated non-Newtonian behavior with increasing shear rates and a propensity to yield with a pronounced flat region. The 400 milligram per milliliter concentration is the closest to the gill raker mucus composition exhibiting a clear change in the state of the mucus from gel-like to a shear thinning fluid after yielding at approximately 0.27 pascals. As outlined in the protocol manuscript, it is important to determine the oscillation strain percentage value within the linear viscoelastic regime of the gill raker mucus before running the dynamic sweeps. Our protocol can be used to ascertain the apparent yield stress of sticky and gel-like biological fluids, and can be extended to tack and peel tests for a full characterization of the adhesivity of mucus-like materials. This protocol paves the way for the hydrodynamic investigation of filter feeding with mucus-like materials, the creation of analytical models, and the advancement of cross-flow and membrane filtration technologies.
发布时间:2020-07-10 JOVE
Our protocol makes it possible to test the sliding of metal for orthopedic implants against articular cartilage and thereby investigating the effects of focal implants or hemiarthroplasty on the biosynthetic activity of particular chondracytes the main advantage of this technique is that it provides a comprehensive insight into both the tribological properties and biologic effects of mechanical loading in the cartilage pairing. This technique might compliment the clinical findings after surgical procedures like implantation of a focal metallic implants to treat an osteochondral defect of the knee or hemiarthroplasty of the hip. Demonstrating the procedure will be Christopher Bauer a postdoc from my laboratory here at the Danube University. Use a commercially available reciprocating tribometer with a cylinder on plate configuration, vertical loading capabilities and adjustable load and sliding speed. Additionally, a liquid cell is required to perform the tests in a lubricating solution. Fix the osteochondral cylinders on the bottom sample holder with the marking aligned with the sliding direction. Begin by determining the contact pressure in the cobalt chromium molybdenum on cartilage system using a pressure measurement film. Place the pressure measurement film at the interface, and apply a static load for 30 seconds to determine initial contact pressure, contact size and shape. Mount the cobalt chromium molybdenum cylinders onto the upper load cell. Add the testing solution into the liquid cell to submerge the osteochondral cylinder and cover the metal cartilage sliding interface. Set testing parameters such as described in normal force stroke and sliding speed which will be maintained throughout the test. Start reciprocal sliding of the metal cylinder against the articular cartilage immersed in the lubricating solution. Monitoring the coefficient of friction throughout the experiment, terminate the experiment. After the desire testing period remove the osteochondral plug from the sample holder rinse it with PBS and store it in medium until further biological analysis. Keep control samples and the testing solution at room temperature for the duration of the test and analyze them together with the samples that have been exposed to mechanical loading. Place, a 24 well plate on a scale and zero it. Rinse the osteochondral plug with PBS and place it in a Petri dish. Then use a scalpel to cut the cartilage from the graft in one piece bisect the cartilage in two equal pieces so that the contact area is equally distributed onto both cartilage pieces and mince one half into approximately one millimeter cubed pieces. Use the second half for gene expression analysis transfer the minced cartilage into one well of the prepared at 24 well plate and determine the tissue weight repeat this process for each sample and add one milliliter of growth medium to each well of the plate. Add 500 microliters of XTT solution to each well and mix then incubate the plate at 37 degrees Celsius and 5%carbon dioxide for four hours after incubation remove the supernatant and transfer it to a five milliliter tube. Extract the tetrazolium product by adding 500 microliters of DMSO to the cartilage tissue in each well and apply continuous agitation for one hour at room temperature, remove the DMSO solution and pull it with the previously collected XTT solution. Transfer 100 microliters of each sample in triplicates to a 96 well plate. Use a plate reader to measure the absorbance at a wavelength of 492 nanometers, and a reference wavelength of 690 nanometers. To isolate the RNA mince the second half of the cartilage tissue obtained from the osteochondral plug into small pieces transfer the tissue to a tube containing ceramic beads and 300 microliters of licensed buffer. With 1%beta mercaptoethanol use a commercial lysate to homogenize the tissue applying 6, 500 RPM for 20 seconds four times with a 20 minute cooling phase after each run. Add 20 microliters of proteinase K and 580 microliters of RNAs free water to each sample, and incubate them at 55 degrees Celsius for 30 minutes. Centrifuge the samples for three minutes at 10, 000 times G and transfer the supernatant to 1.5 milliliter tubes. Add 0.5 volumes of 90%ethanol to each tube and mix then transfer 700 microliters of the sample to an RNA binding column in a two milliliter collection tube and centrifuge it at 8, 000 times G for 15 seconds. Discard the flow-through and repeat the centrifigation step for the rest of the lysate. Add a 350 microliters of buffer RW one to the column. Centrifuge it at 8, 000 times G for 15 seconds and discard the flow-through. Mix 10 microliters of DNA stock solution and 70 microliters of buffer RDD. Add the solution to the RNA purification membrane and incubate it at room temperature for 15 minutes then add 350 microliters of buffer RW one to the column. Centrifuge it at 8, 000 times G for 15 seconds and discard the flow-through add 500 microliters of buffer RPE to the RNA purification column and Centrifuge it at 8, 000 times G for 15 seconds discard the flow through and to add another 500 microliters of buffer RPE to the RNA purification column then centrifuge it at 8, 000 times G for two minutes place the column in a new 1.5 milliliter collection tube and add 30 microliters of RNAs free water centrifuge the tube at 8, 000 times G for one minute, to allude the RNA synthesized cDNA using a commercial kit thaw and mix all regions, add the RNA sample and perform the reaction and the thermal cycler as described in the text manuscript. Add nine microliters of RTQ PCR master mix, and one microliter of cDNA to each well of a 96 well plate with each sample in triplicates glows the PCR plate with ceiling oil and centrifuge it at 877 times G for 10 minutes at four degrees Celsius perform RTQ PCR and a precision and thermal cycler. According to manuscript directions. Prior to testing the contact area and contact pressure at the metal cartilage interface must be confirmed using a pressure measurement film. Physiological loading condition can then be confirmed by comparing the obtained imprint with reference inference for defined contact A low friction coefficient can be maintained for at least one hour with a migrating contact area. The extracellular matrix composition and structure can be determined with saffron and O staining. The intensity of saffron and O staining is proportional to the proteoglycan content. The proteoglycan content varies over the articular surface but should be uniform throughout the tissue in baseline samples, show extraction of glycosaminoglycans which can be counteracted by mechanical loading. Metabolic activity of the bovine articular chondracytes is independent of the harvesting site but shows an increase with mechanical loading. The gene expression levels of cartilage specific genes increased with physiological loading conditions. Whereas catabolic genes are up-regulated with stationary contact area. Following this procedure, additional analysis can be performed including determination of cartilage web products and analysis of articular surface using scanning electron microscopy and furthermore we try to optimize lubrication of articular cartilage in various tribological pairings.
发布时间:2020-05-14 JOVE
The two-phase solid-liquid fabrication can also be applied to the manufacturing of structural microsphere materials in various field of study, including the electronics, biopharmaceutical, energy, and defense sectors. This system does not require wires or electrical connection and allows a wide range of applications related to microstructure deformation to be measured. Before beginning the procedure, construct an experimental platform that includes a modified 3-D printer, a strain gauge indicator, a driving device, a support frame, an aluminum bar, a PDMS lens, a smartphone, weights, a printed amplifier, and a strain gauge. Set the height of the nylon layer in the printer to 0.05 millimeters. Set the diameter of the printing head to 0.2 millimeters, and set the nozzle temperature to 220 degrees Celsius. Set the printing speed to 2000 millimeters per minute. Adjust the orientation of the spherical extrusion head so that the metal nozzle faces the low temperature platform and print a contour to ensure a normal extrusion. Then hang the nylon on the column. The front end must enter the printing coil container to be melted by the metal nozzle. To assemble the PDMS microscope, use a magnetic stirrer to mix a 10 to one weight ratio of PDMS precursor to curing agent solution and de-gas the mixture for 40 minutes. When all of the bubbles have been removed, pour the mixture into the PDMS container of the spherical extrusion head and rotate the spherical extrusion head and platform so that the plastic nozzle faces the high temperature platform. Set the plastic nozzle increment to 50 microliters and use the nozzle rotation and the stepper motor in the Z-axis to place the bottom end of the pipette device 20 millimeters away from the mold. Then heat the high temperature platform and squeeze the PDMS container to print the PDMS lens. When the printed PDMS lens has cooled to room temperature, use rubber tweezers to remove it from the printer. To perform a loading test strain measurement, use nuts and bolts to fix one end of a 380 by 51 by 3.8 millimeter aluminum 6063-T83 bar to the operating table and draw a cross at the center and 160 millimeters from the free end of the cantilever beam. To remove the oxide layer on the beam, polish the surface with fine sandpaper at an about 45 degree angle from the direction of the strain gauge wire grid. Use cotton wool soaked in acetone to wipe the surface of the sanded cantilever beam and the surface of the strain gauge paste. Then connect the driving device and the strain gauge indicator and turn on the power. Next, mount a strain gauge onto the center surface of the aluminum bar at its fixed end and fix a standard weight to the free end of the cantilever beam to control the concentrated force input. Record a baseline read-out using a conventional strain gauge indicator with a quarter bridge connection method before replacing the strain gauge with a nylon amplifier. Attach the PDMS lens onto a smartphone camera with an eight megapixel sensor at a focus distance of 29 millimeters and adjust the focal length of the camera until a clear image is obtained. Then use the PDMS microscope to read the displacement of the pointer. To perform a finite element analysis, import the cantilever beam and the amplifying mechanism into the material library of the software and simulate their placement positions. Analyze the mechanical properties of the amplifying mechanism pointer under the action of a cantilever beam and use tetrahedral elements with a fine element size to generate meshes for use in 3-D geometric models. Then refine the flexure hinges, especially the hinge between the pointer and the other bodies, and apply a concentrated force of one newton to the center of the free end of the cantilever beam. As the platform temperature is increased, the droplet diameter and curvature radius decrease, and the contact angle increases. Here a comparison of the experimental displacement measurement with the FEA simulations for nylon is shown, while this graph illustrates the minimum and maximum discrepancies between the slopes for ABS. In this representative experiment, the measurement sensitivities for nylon and ABS were determined. Controlling the molding temperature of the PDMS lens is difficult. We use a non-contact infrared radiation thermometer and high-temperature platform to ensure that the temperature changes are within tolerance. This solid-liquid manufacturing method can also be applied to studies in the biopharmaceuticals field, particularly for the preparation of microsphere structures.
发布时间:2020-01-30 JOVE
When charging aluminum alloys with hydrogen, the presence of oxide film on the surface is a challenge. To solve this problem, we have developed a method that can introduce high amount of hydrogen into aluminum alloys using friction in water. The method is easiest to understand when the rotation of the stir bar and the specimen on the polishing paper can be visualized. Success depends on stabilizing the rotation of the stir bar. Demonstrating the procedure will Ms.Michiko Arayama, an undergraduate student from my laboratory. Fabricate the aluminum alloy test pieces as described in the text. Perform a solution heat treatment by heating the test pieces in an air furnace at 520 degrees Celsius for one hour and then quenching the test pieces in water. For the next step, the peak-aging heat treatment, anneal the test pieces at 175 degrees Celsius for 18 hours. The final step in preparing the test pieces is polishing the surface using silicon carbide emery paper. Before proceeding to the friction in water procedure, weigh and measure the test pieces. Use an electric balance to weigh the test pieces to a precision of 0.0001 grams. Use an optical comparator to measure the thickness and width of the test pieces to a precision of 0.0001 millimeters. The friction in water procedure is carried out in a magnetically stirred custom-made reaction vessel. To begin, use glue to attach two test pieces to a fluorocarbon polymer triangular stir bar. Next, prepare the reaction vessel, a custom-made glass container. Use double-sided tape to attach polishing paper to the inside bottom of the reaction vessel. Place the reaction vessel on the magnetic stirrer. Then place the test pieces and stir bar on top of the polishing paper and the reaction vessel, and add 100 milliliters of distilled water. Place the rubber cover on the reaction vessel. Connect the gas inlet to high-purity argon, and turn on the gas. Connect the gas outlet to a gas chromatograph. Inset the pH probe into the vessel through the rubber cover. Turn on the argon. Once the gas in the reaction vessel as been completely replaced by the argon, the apparatus is ready for charging the aluminum alloy test pieces. Turn on the magnetic stirrer. To stabilize movement of the stir bar in the water, controlling the rotation speed is important. A speed ranging from 60 rpm to 240 rpm is best. Every two minutes, measure the hydrogen concentration using the gas chromatograph, and measure the pH. After one hour, turn off the magnetic stirrer, and remove the test pieces and stir bar from the reaction vessel. To detach the test pieces from the stir bar, immerse them in acetone, and apply ultrasonic vibration for five minutes. Before proceeding to the next step, measure the weight and thickness of the test pieces. Use a tensile test machine to measure the material properties of the test pieces. Set the crosshead speed of the machine to two millimeters per minute. Then measure the stress-strain relationship for the test pieces. To calculate the amount of hydrogen absorbed during the friction in water procedure, first measure the hydrogen released by the test pieces when heated. Cut the test piece to a rectangular shape of one by five by 10 millimeters. Place the test piece inside a quartz tube with a diameter of 10 millimeters, and place the quartz tube in a tubular furnace. Connect the tube to the gas chromatograph and to the argon gas supply. Turn on the flow of argon gas. Heat the quartz tube containing the test piece, increasing the temperature at a constant rate of 200 degrees Celsius per hour until it reaches a temperature of 625 degrees Celsius. While the quartz tube and test piece are being heated, use the gas chromatograph to measure the hydrogen released every two minutes. Aluminum-magnesium-silicon alloys with three different iron concentrations were subjected to the friction in water procedure, 0.1%iron, 0.2%iron, and 0.7%iron. Regardless of the iron concentration, the test pieces emitted large amounts of hydrogen during the procedure. Thermal desorption analysis was performed on both uncharged and hydrogen-charged samples. Regardless of the iron concentration of the alloy, the total hydrogen concentration increased as a result of the friction in water procedure. Compared to the method of pre-stained in humid air, friction in water is an effective method of hydrogen charging an aluminum alloy. Thermal desorption analysis showed a higher hydrogen release rate for alloy charged using the friction in water procedure, and the calculated hydrogen concentration was substantially higher. Compared to uncharged alloy samples, hydrogen-charged alloy samples showed lower ductility. This indicates that the friction in water procedure resulted in hydrogen embrittlement. Secondary electron microscopy was used to examine the fracture morphology of aluminum-magnesium-silicon alloy containing 0.1%iron. After the friction in water procedure, the morphology changed to a grain boundary fracture. This indicates that hydrogen atoms introduced by the friction in water procedure enhanced the decohesion of grain boundaries, leading to hydrogen embrittlement. It is possible to hydrogen-charge aluminum alloys through exposure and humid air with brittle deformation with a slow stirring rate. However, the current method result in a greater amount of hydrogen charging. This method makes it easy for researchers to evaluate the hydrogen embrittlement sensitivity of aluminum alloys that have a variety of different chemical compositions. It may be applied to the development of hydrogen storage materials.
发布时间:2020-01-28 JOVE